
Dynamic Data Analysis – v5.12.01 - © KAPPA 1988-2017
Chapte
r 2 – T heory- p17/743
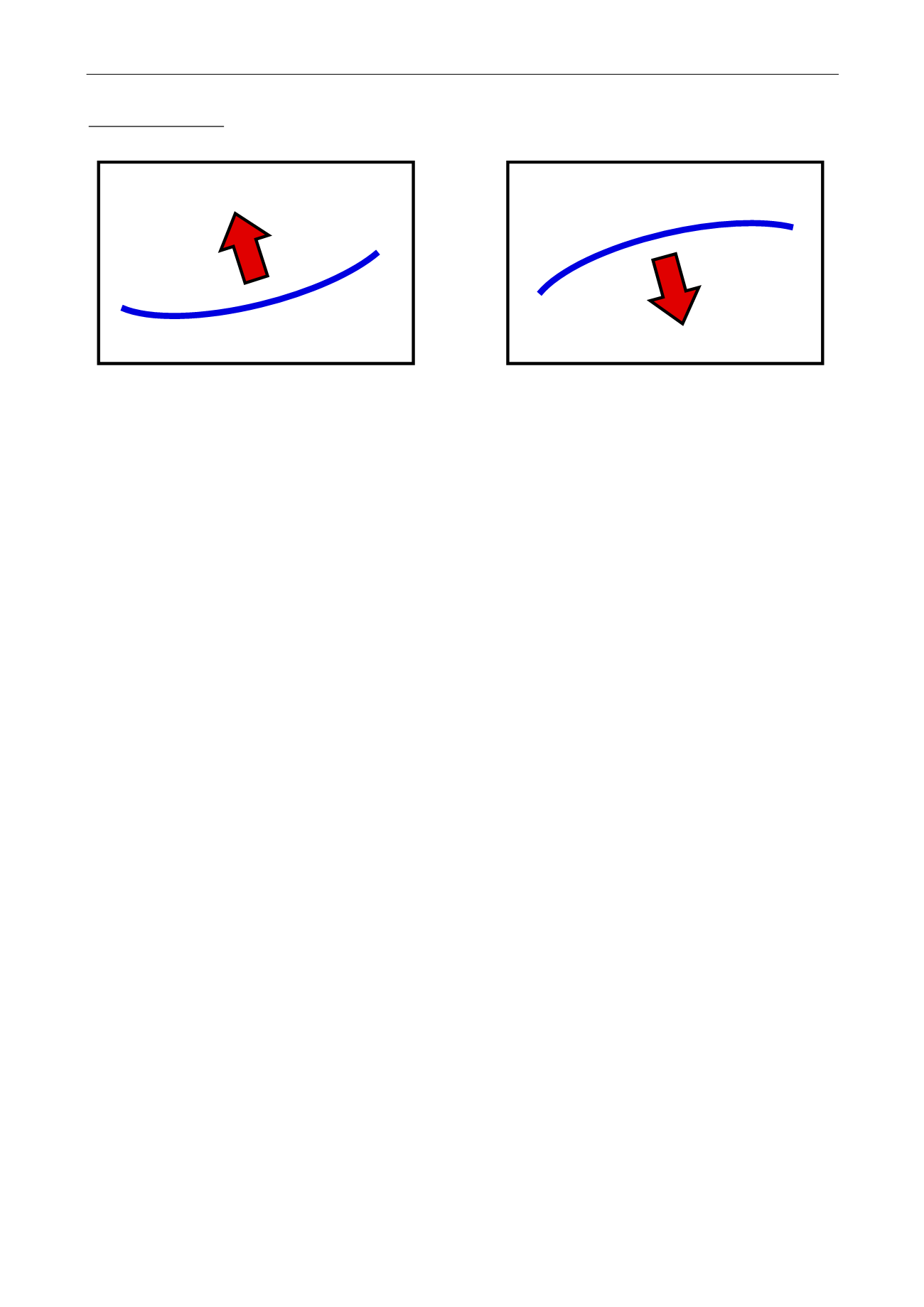
Physical meaning: If we look at the last relation and consider the flow in only one direction:
If the curvature of the pressure profile is positive, the pressure will locally increase. If the
curvature is negative the pressure will decrease. The speed of the pressure change, in
whatever direction, will be proportional to this curvature.
If the permeability is large, the pressure change will be fast. Basically, the more permeable
the formation, the quicker the formation fluid will react to a local pressure disturbance.
If the viscosity is large, the pressure change will be slow. Basically, the more viscous the
fluid, the slower the formation fluid will react to a local pressure disturbance.
The ratio k/
, to which the speed of reaction is proportional, is also called the mobility.
If the porosity is large, the pressure change will be small, and therefore, at a given time,
relatively slow. Basically, the more porous the formation, the lower the pressure change
that will be required to produce / receive the same mass of fluid.
If the total compressibility is large, the pressure change will be small, and therefore, at a
given time, slow. Basically, the more compressible the formation is, the lower the pressure
change required to produce / receive the same mass of fluid.
The term 1/
c
t
, to which the amplitude of the reaction is proportional, is also called the
storativity.
Mobility and productivity seems to play the same role in the diffusion equation. However
the mobility will also be found in the inner boundary conditions and this is where the role of
the different parameters will diverge.
Generally, the diffusion equation used in most analytical models is the radial formulation. The
reference point of such a radial solution is the producing or injecting well. This system of
coordinates is well suited to model radial flow to and from the well. In the following we will
stay in radial coordinates.
The diffusion equation shown above describes the flow of what we call a homogeneous
reservoir where this same, unique equation will be applied everywhere in the reservoir. More
complex reservoirs can be modelled, and this diffusion equation will be replaced by
formulations involving different pressures at the same point (double-porosity, double-
permeability reservoirs) or changing diffusivity and mobility in different locations (composite
reservoirs). The different reservoir models are detailed in the chapter on the subject.
















