
Dynamic Data Analysis – v5.12.01 - © KAPPA 1988-2017
Chapte
r 2 – T heory- p16/743
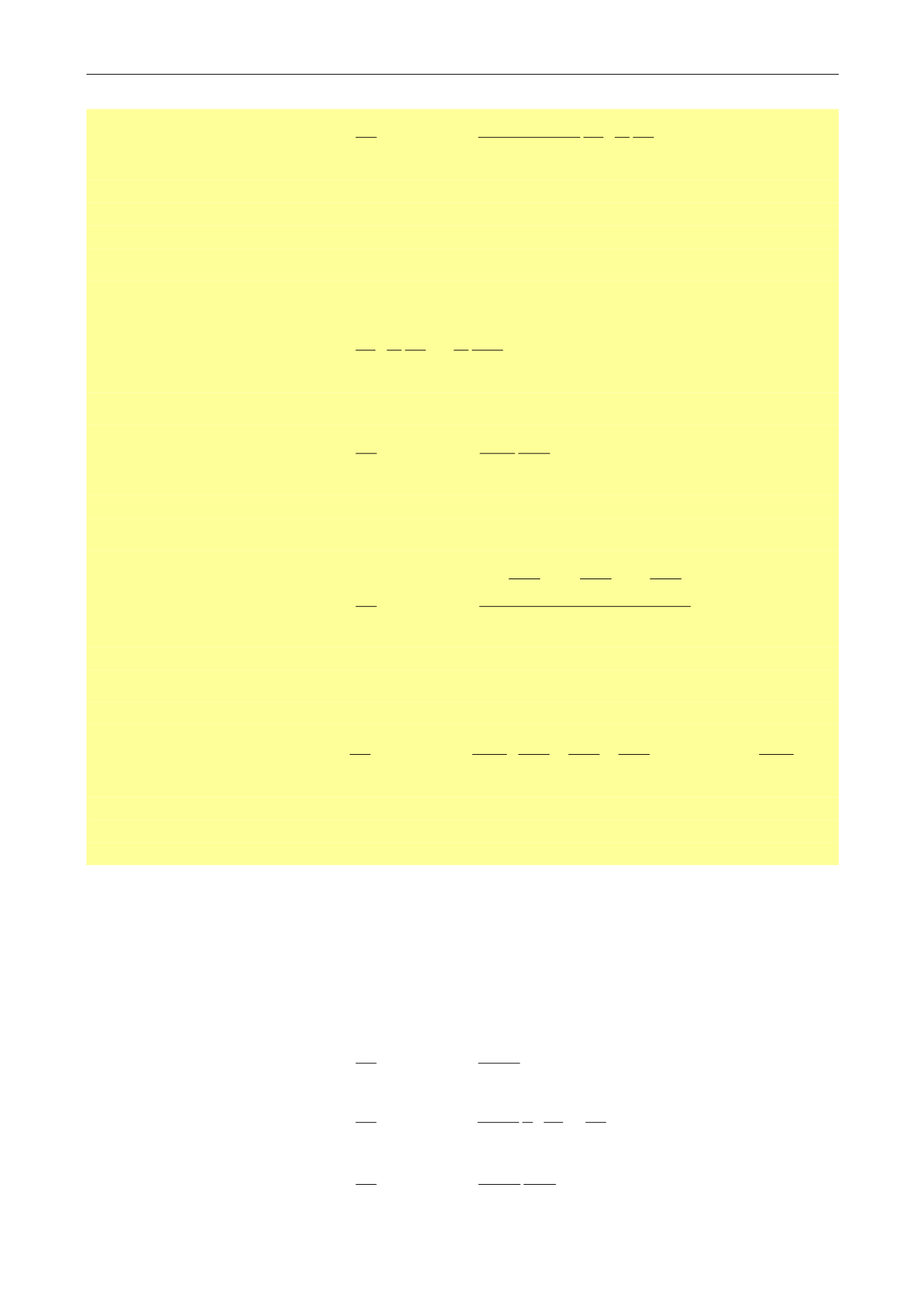
New differential form:
x
p
x
c c
k
t
p
fluid
f
x
0002637
.0
The last equation required is PVT related, in order to assess the relation between the fluid
compressibility and the pressure. The simplest equation that can be used is the
slightly
compressible fluid
, assuming that the fluid compressibility is constant, i.e. independent of
the pressure. So we get a total constant compressibility:
Slightly compressible fluid:
fluid
f
t
c c c
Constant
Also we can consider that:
2
2
x
p
x
p
x
We finally get the diffusion equation in the x direction:
Diffusion equation; direction x:
2
2
0002637
.0
x
p
c
k
t
p
t
x
The process above was dealing with the flux in only one direction. If we consider now the flux
through an arbitrarily small cube in all three directions, we get:
Generic diffusion equation:
t
z
y
x
c
z
p
k
y
p
k
x
p
k
t
p
2
2
2
2
2
2
0002637
.0
If we consider an isotropic reservoir, all permeability components are equal to a unique
permeability, k:
Isotropic diffusion equation:
p
c
k
z
p
y
p
x
p
c
k
t
p
t
t
2
2
2
2
2
2
2
0002637
.0
0002637
.0
The operator on the right end side is called the Laplace operator, or Laplacian. It is also written
∆p, but we will avoid this form in order not to cause confusion with the pressure change in
time, also noted ∆p.
2.A.3
Diffusion in a homogeneous isotropic reservoir
When combining the law of conservation of mass, the simplest pressure gradient equation
(Darcy’s law) and the simplest material balance relation (slightly compressible fluid
assumption), we get the simplest version of the diffusion equation, given below in three
different forms:
General form:
p
c
k
t
p
t
2
0002637
.0
Radial flow:
r
p
r
r rc
k
t
p
t
1
0002637
.0
Linear flow:
2
2
0002637
.0
x
p
c
k
t
p
t
















